7th Grade Math Worksheets Proportions
This blog post features a comprehensive collection of 7th-grade math worksheets focused on proportions. Whether you are a middle school student, parent, or teacher, these worksheets provide an engaging way to reinforce the fundamental concepts of proportions. With a variety of problem-solving activities and step-by-step explanations, these worksheets offer a valuable resource for students to enhance their understanding of this important math topic.
Table of Images 👆
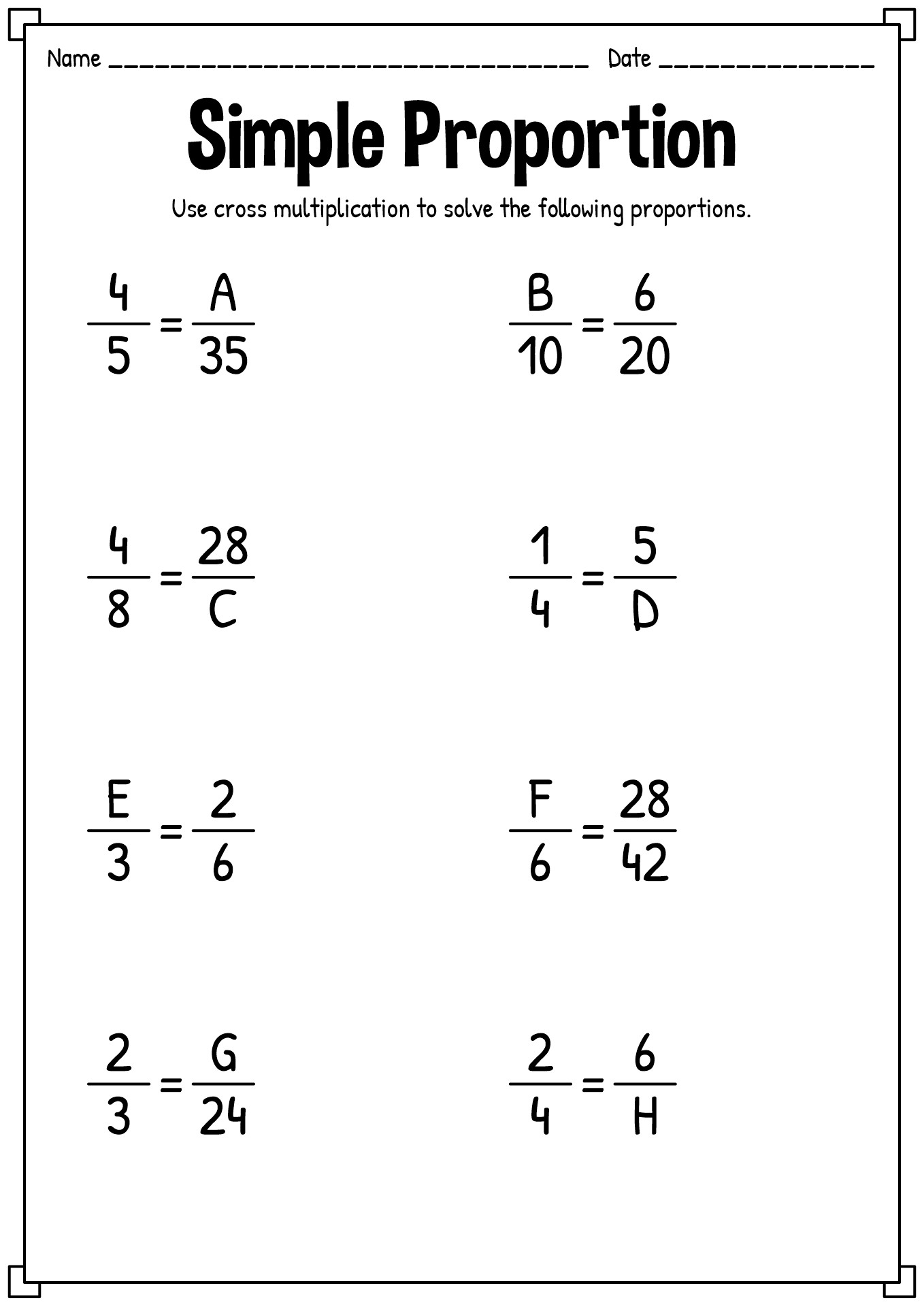
- Proportions Worksheets 7th Grade
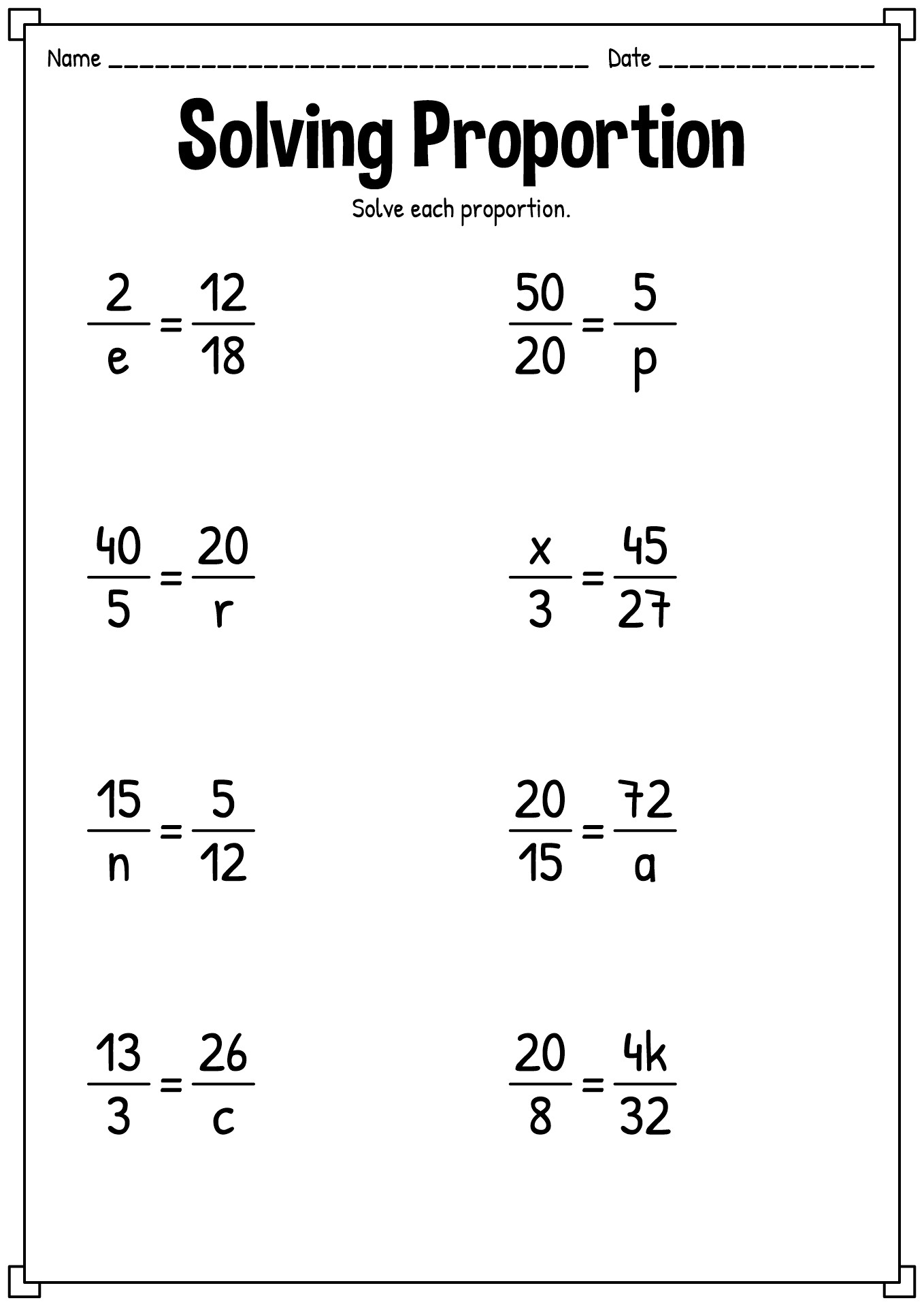
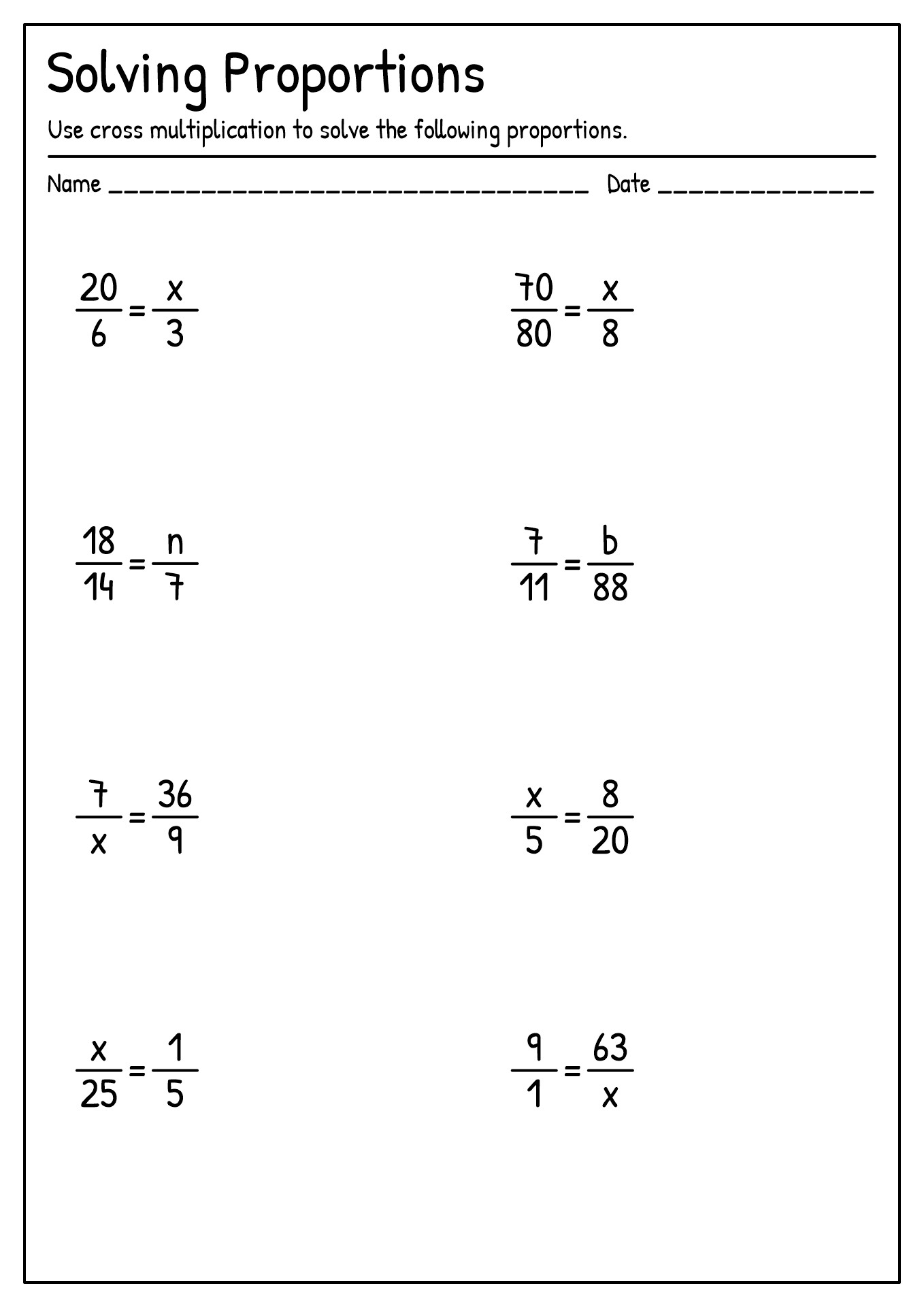
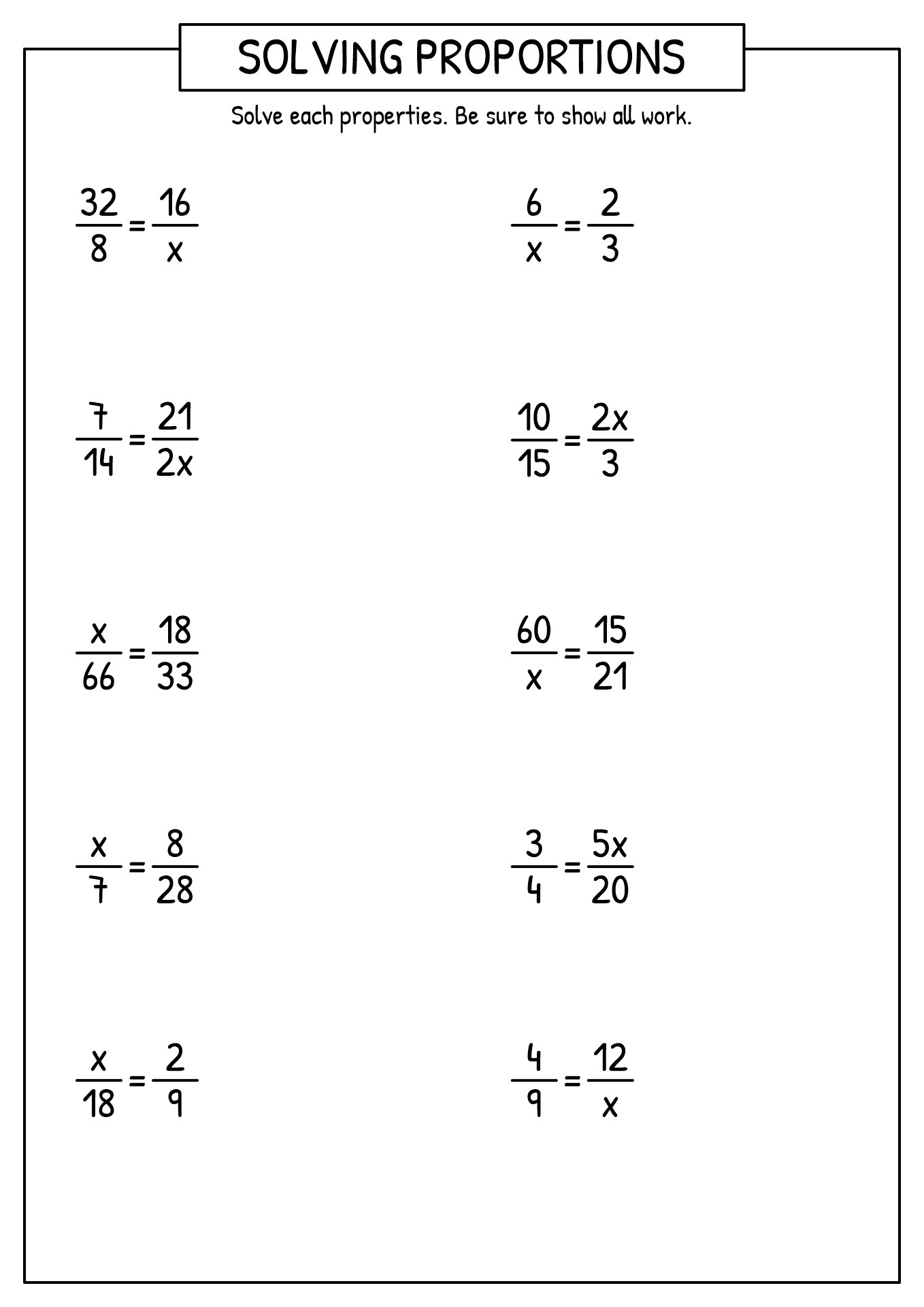
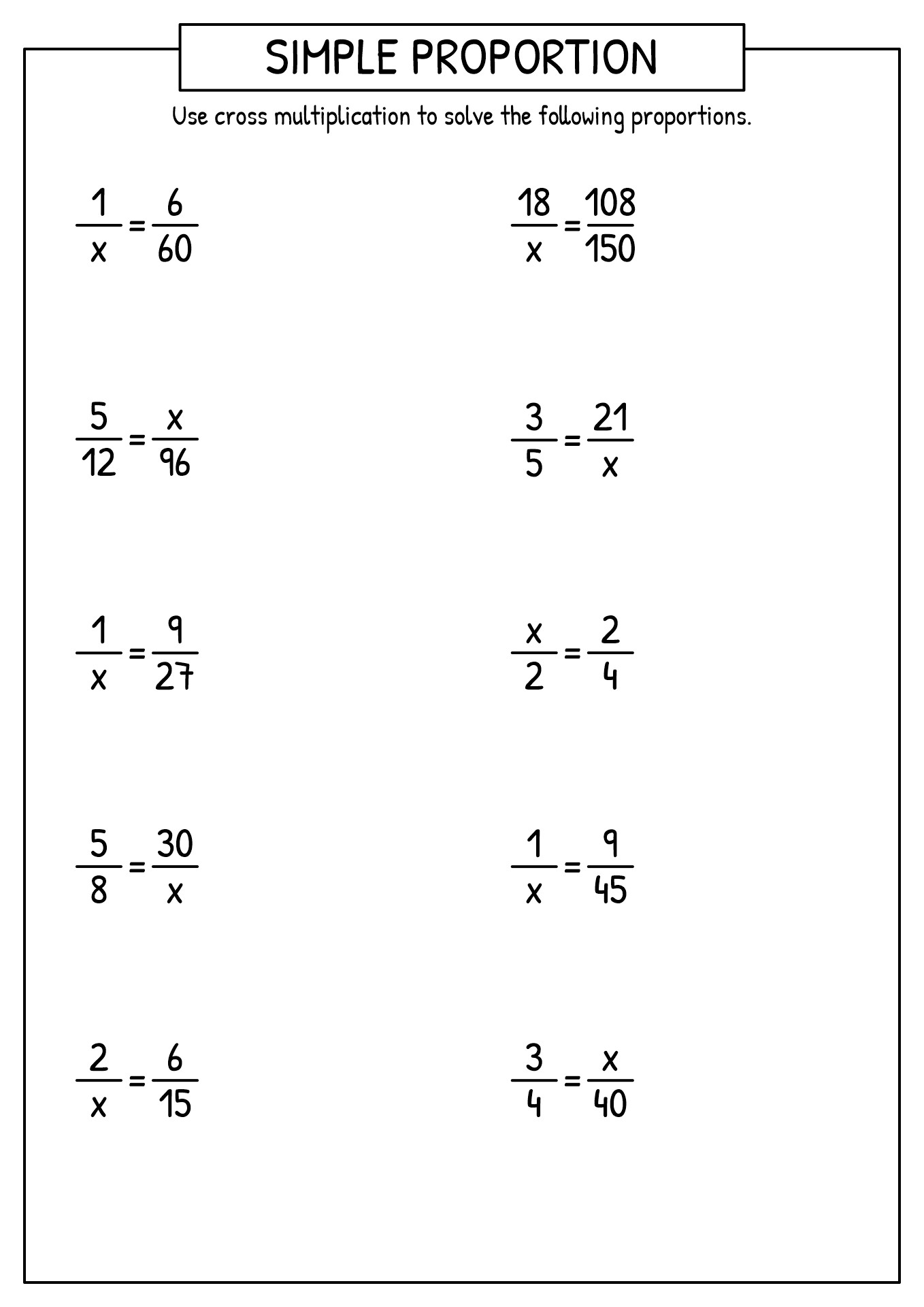
- Solving Proportions Worksheet
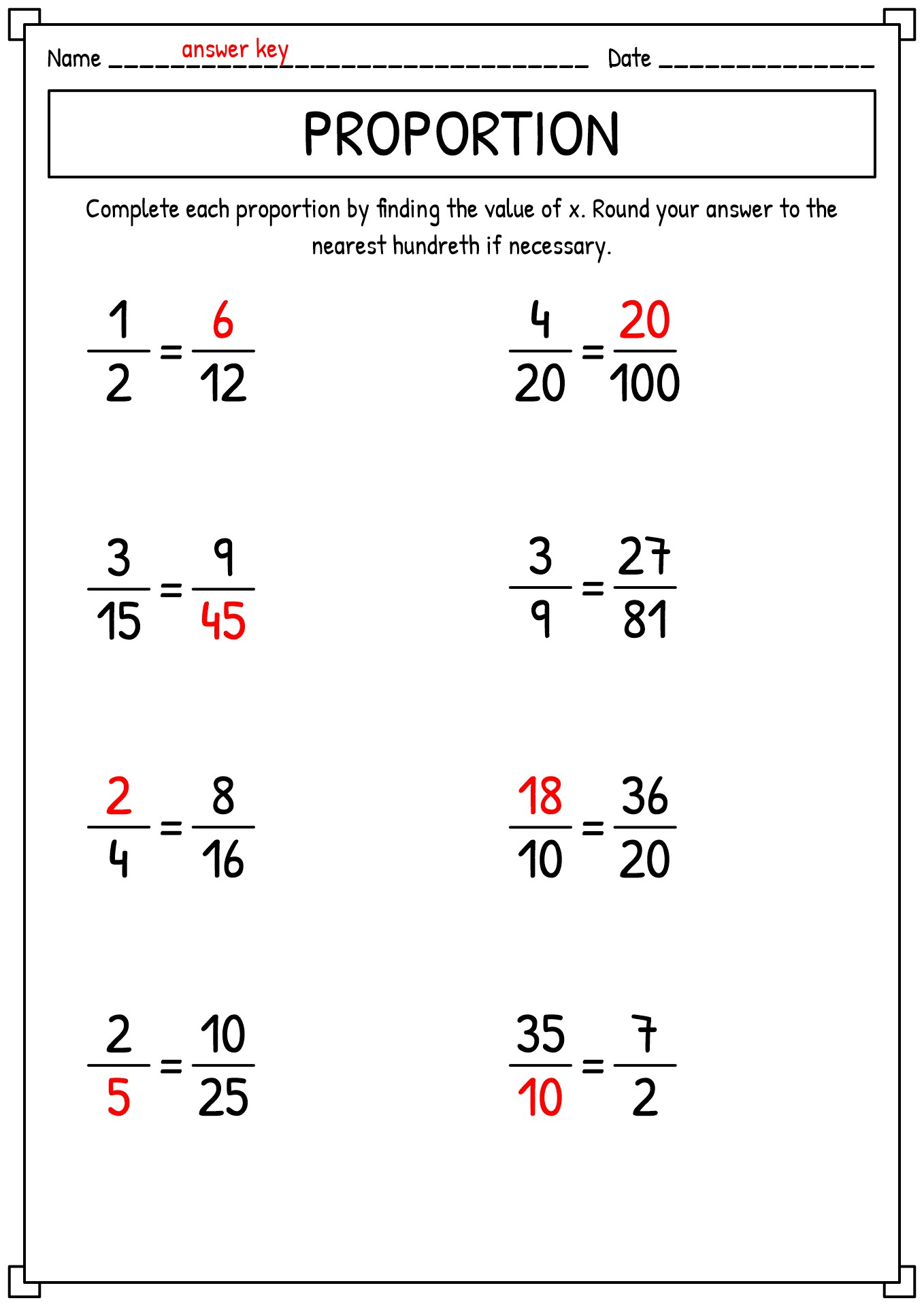
- 7th Grade Proportions Worksheet Answers
- 7th Grade Math Worksheets
- 6th Grade Math Ratio Worksheets
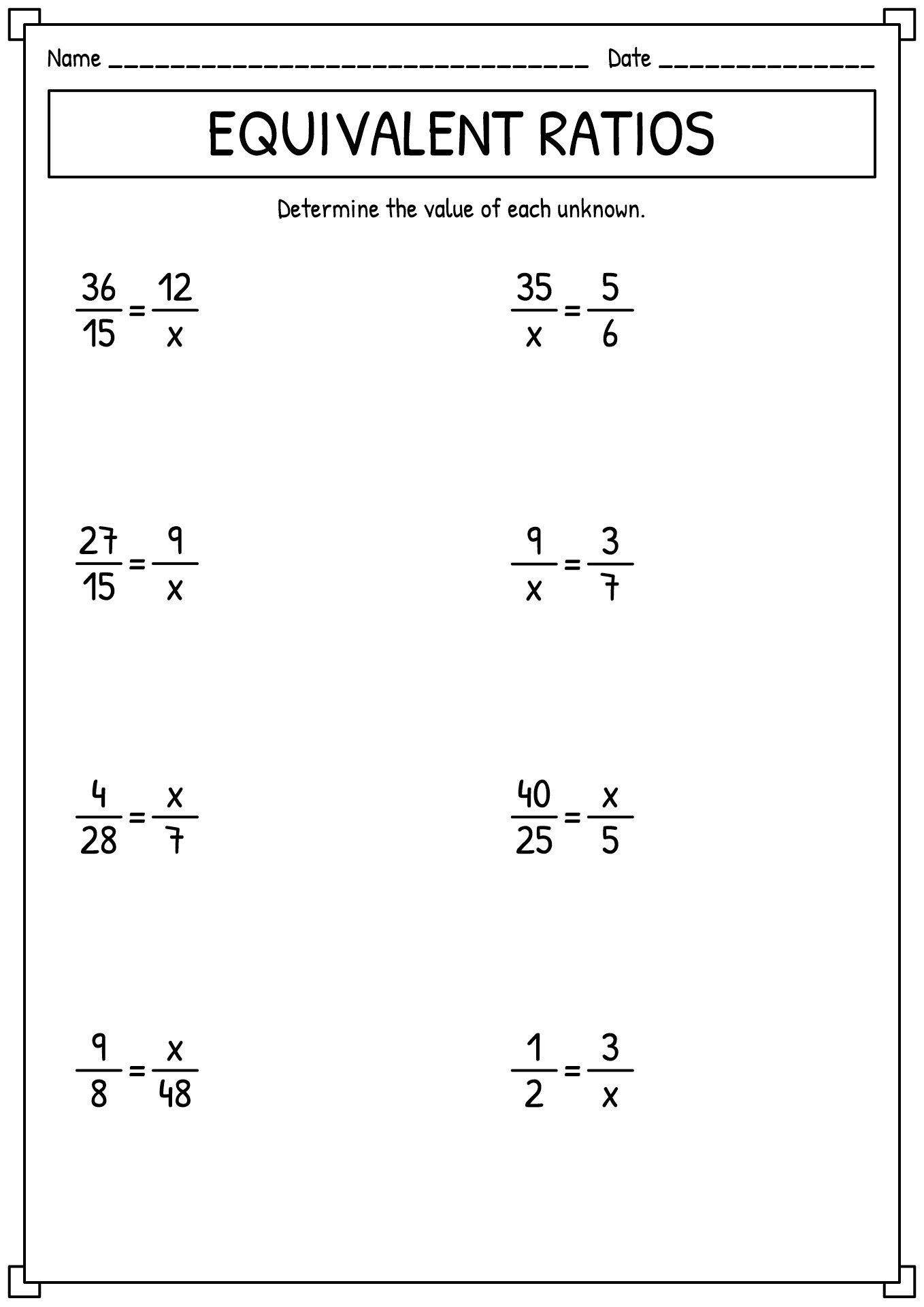
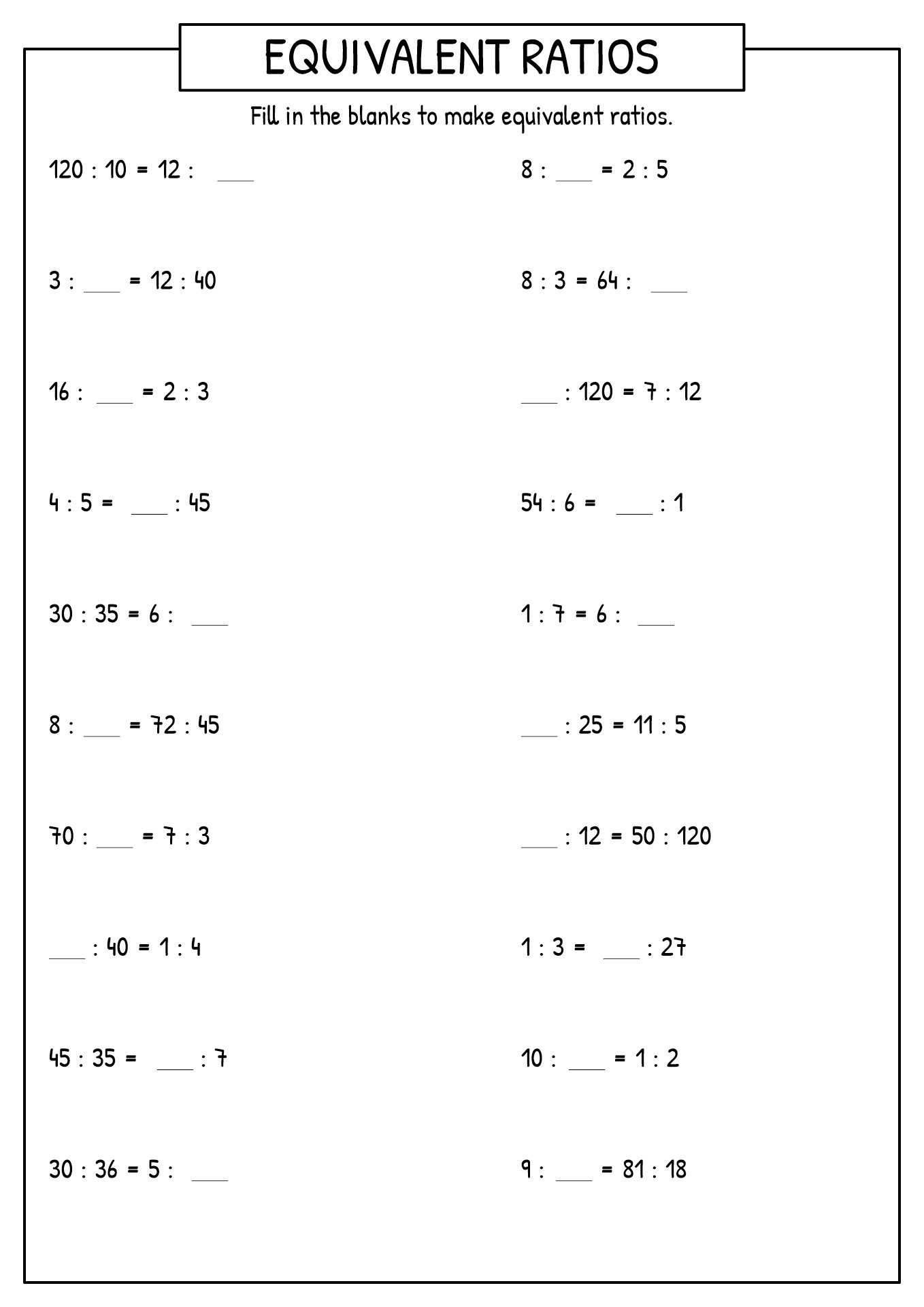
- 7th Grade Equivalent Ratios Worksheet
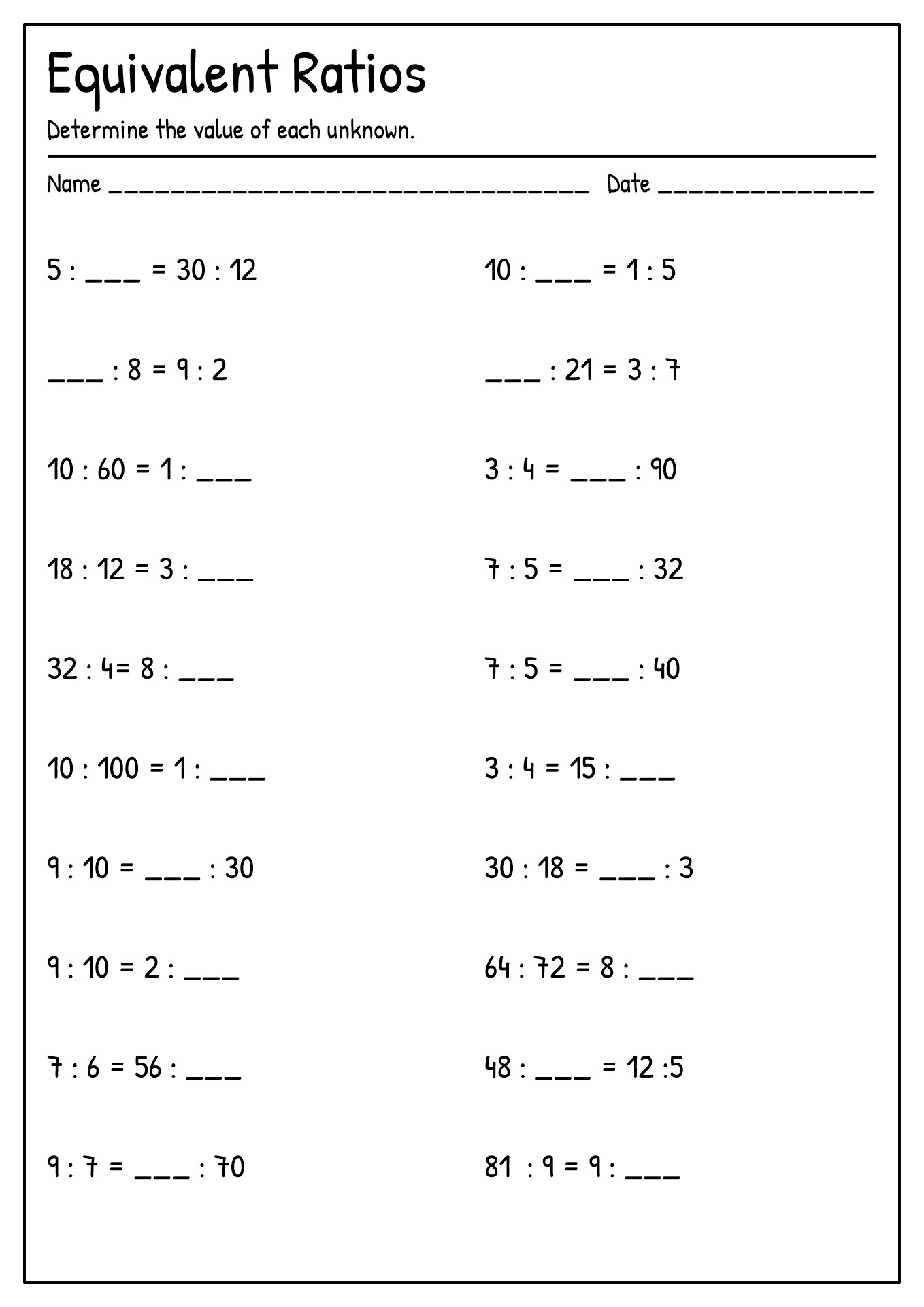
- Solving Ratios and Proportions Worksheets
- 7th Grade Math Worksheets Integers Printable
- 6th Grade Ratio Worksheets
- Ratio and Proportion Worksheets
- Equivalent Ratios Worksheets
- 7th Grade Ratio Word Problems Worksheets
14 Images of 7th Grade Math Worksheets Proportions
Proportions Worksheets 7th Grade
Solving Proportions Worksheet
7th Grade Proportions Worksheet Answers
7th Grade Math Worksheets
6th Grade Math Ratio Worksheets
7th Grade Equivalent Ratios Worksheet
Solving Ratios and Proportions Worksheets
7th Grade Math Worksheets Integers Printable
7th Grade Math Worksheets
6th Grade Ratio Worksheets
Solving Proportions Worksheet
Ratio and Proportion Worksheets
Equivalent Ratios Worksheets
7th Grade Ratio Word Problems Worksheets
More 7th Grade Worksheets
7th Grade Vocabulary WorksheetsPre-Algebra 7th Grade Math Worksheets
7th Grade Math Worksheets Proportions
Complex Sentence Worksheets 7th Grade
Geometry Angles Worksheet 7th Grade Math
Learn how to solve proportion problems with these 7th Grade Math Worksheets Proportions!
Summary: A proportion is an assertion that two ratios are equivalent. Proportion is an equalization that explains two ratios are equivalent because they have the same values. It defines the parallelism of the ratios. We can write proportions as equivalent fractions (a/b = c/d) or equal ratios (a : b = c ; d). Understanding the concept of ratios and proportions is imperative because it is the foundation of various mathematic and scientific ideas and conceptions.
What is Proportions in Math?
In the mathematical world, a proportion is an assertion that two ratios are equivalent. We can write it as equivalent fractions (1/4 = 5/20) or equal ratios (1 : 4 = 5 : 20). The term "equivalent" means we can multiply or divide one scale by another fixed value to create the other. Based on the previous example, we could multiply 1:4 by five (5) or divide 5/20 by 5.
1 : 4 = (1 x 5) : (4 x 5) = 5 : 20
5 : 20 = (5 ÷ 5) : (20 ÷ 5) = 1 : 2
Based on the example, we can conclude the ratios are proportional since the factor of five (5) link the ratios. There are four terms of proportion (a/b = c/d or a:b = c:d). The a and d are "extremes", and b and c are "means". In proportional ratios, the results of the means are the same as the extremes' results. Based on the above example, the results of (1 x 20) and (4 x 5) are the same, 20. Mastering how to solve proportions will help students to prepare themselves for algebra.
What is Ratio in Math?
According to Khan Academy, the ratio is a comparison of two elements. We can write it as b/c or b:c. The "a" is the antecedent (prior), and the "b" is the consequent (later). A real-life example of using ratio is when you want to make double portions of a recipe. In mathematics, "equivalent ratio" means a ratio with an identical value. Ratios appear to help humans make a comparison. We can shorten them to the minimum to achieve simplicity. We can write ratios in two techniques, part to part or part to whole. To write it, you should pay attention to these details:
- Settle whether it is part of part or part to whole.
- Decide the "part" and the "whole".
- Put values to the ratio.
- Shorten them into an integer-to-integer rate.
How to Teach Proportions?
Without realizing it, we often apply the proportions in our daily life. Hence, learning about them is necessary for the students. The teacher should give the students a fun teaching experience for the students. A proper learning strategy would improve the student's understanding of the material. Based on David Ben-Chaim from the Israel Institute of Technology, the teacher should ensure the teaching should include theoretical and practical knowledge for balance learning. This teaching strategy will allow the students to learn mathematics in a real-life context and offer creativity and teaching freedom to the teachers.
Teachers can use the "equivalent rates table" to introduce the students to proportional reasoning. Give the students easy numbers first and demonstrate a real-life example to make them understand easier. As the students get used, the teachers can level up the tables. After mastering the tables, the students can practice with the word problems. Assign them the problems and let them think and discuss them. This learning progress will help them to prepare for facing algebra.
Why Should We Learn Proportions?
In mathematics, fractions are the foundation of ratios and proportions. When we write a fraction as a:b, it is a ratio. Proportions are a situation where two ratios have the same value. Understanding the concept of ratios and proportions is imperative because it is the foundation of various mathematic and scientific ideas and conceptions. In real-life, ratios and proportions are common mathematical knowledge we can find easily. For example, we can use ratios to write a comparison of something. Proportions are necessary if we want to draw an accurate image of something. It also helps when we deal with money in business.
What are the Types of Proportions?
Proportion is an equalization that explains two ratios are equivalent because they have the same values. It defines the parallelism of the ratios. There are three categories of proportions, direct, inverse, and continued proportion. Direct proportions explain the link between two numerical elements, which means the increase in one ratio will affect the other. These rules also apply when the quantity decrease. Inverse proportions define the connection between two ratios in which the growth of one side will lead to the reduction of the other. The same rules apply to the opposite as well. The decline of one part will affect the increase of the other. The continued proportions require us to convert the "means" to a singular number. For example, the first ratio is (ca : bc), and the second is (bc : bd). The results of the continued proportion would be "ca : bc : bd".
Have something to share?
Who is Worksheeto?
At Worksheeto, we are committed to delivering an extensive and varied portfolio of superior quality worksheets, designed to address the educational demands of students, educators, and parents.
Comments
I found the 7th Grade Math Worksheets Proportions very helpful and well-organized. It provided clear explanations and engaging exercises to reinforce my understanding of proportions. Highly recommended!
The 7th Grade Math Worksheets Proportions resource is helpful and engaging, providing a great way for students to practice and understand proportional relationships. It offers clear explanations and a variety of exercises that make learning math enjoyable.
Great resource to reinforce proportional reasoning skills in 7th grade math! The worksheets are clearly organized and provide a helpful variety of practice problems. Thanks for making learning math enjoyable!
I found the 7th Grade Math Worksheets Proportions to be a helpful resource for my child. The worksheets were clear and easy to follow, facilitating their understanding of proportions. Thank you for providing such a useful and educational tool!
This printable resource on 7th Grade Math Worksheets Proportions is a valuable tool for reinforcing key math concepts. It provides clear and concise practice problems that will help students develop a strong understanding of proportions. Highly recommended for anyone looking to improve their math skills!
I found the 7th Grade Math Worksheets Proportions very helpful in reinforcing my understanding of proportions. The worksheets were clear and easy to follow, making it enjoyable to practice and improve my math skills. Thank you!