Integral U-Substitution Worksheet

Are you a student studying calculus and looking for practice problems to master the technique of integral u-substitution? Look no further! In this blog post, we have created a comprehensive worksheet that focuses on this essential topic. The u-substitution method allows you to simplify and solve complex integrals by making a strategic substitution for the variable. With the help of this worksheet, you will have ample opportunities to practice identifying the suitable u-substitution, applying the appropriate formula, and finding the final solution. So, let's dive in and sharpen our skills in integral u-substitution!

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

  Calculus U-Substitution Examples

---

What is the purpose of using u-substitution in an integral?

The purpose of using u-substitution in an integral is to simplify the integrand by replacing it with a new variable, u, that will make the integration easier. This technique allows for reducing complicated integrals into simpler forms that can then be easily solved using standard integration methods. By cleverly choosing u as a function of the original variable and its derivative, the integral can be transformed into a more manageable form, making the calculation more straightforward.

How do you choose the appropriate substitution for a given integral?

When choosing the appropriate substitution for a given integral, look for a part of the integrand that resembles the derivative of another function that is present in the integral. Common substitutions involve trigonometric functions, algebraic functions, and exponential functions. Additionally, consider simplifying the integrand before choosing the substitution to make the integration process easier. Practice and familiarity with different types of substitutions will help in effectively selecting the appropriate one for a given integral.

What steps are involved in carrying out u-substitution?

To carry out u-substitution, the steps involve selecting a suitable u to substitute for a complicated expression in the integrand, calculating du (the derivative of u with respect to x), rewriting the integral in terms of u and du, integrating with respect to u, and finally replacing u with the original expression in terms of x to obtain the final result of the integral.

Can you provide an example of a basic integral that requires u-substitution?

Sure! An example of a basic integral that requires u-substitution is ?2x(x^2 + 1)^4 dx. To solve this integral, we can let u = x^2 + 1. Then, du = 2x dx, which can be rearranged as 1/2 du = x dx. Substituting u and 1/2 du back into the integral gives us ?u^4 * (1/2) du = 1/2 ?u^4 du. Finally, integrating with respect to u gives us 1/2 * (u^5 / 5) + C, where C is the constant of integration. Substitute back u = x^2 + 1 into our answer to get the final result of (x^2 + 1)^5 / 10 + C.

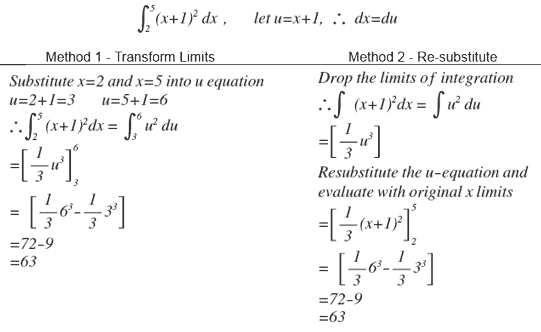
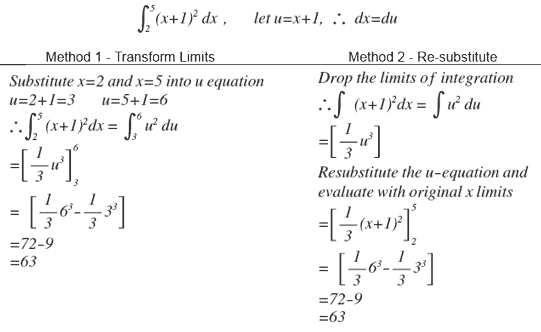
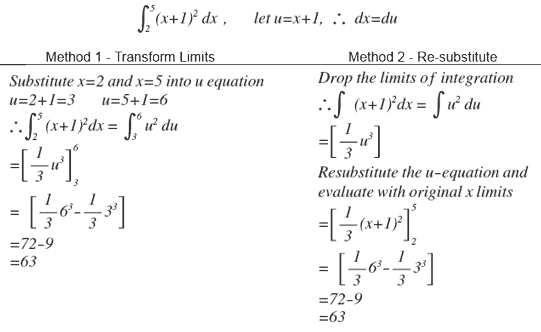
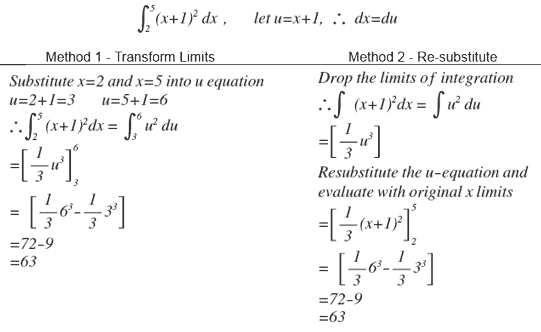
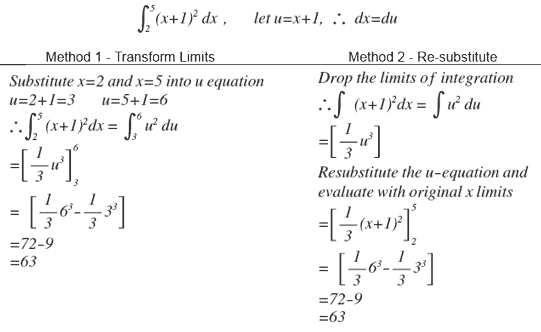
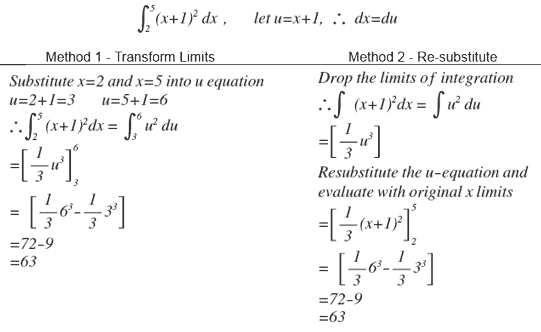
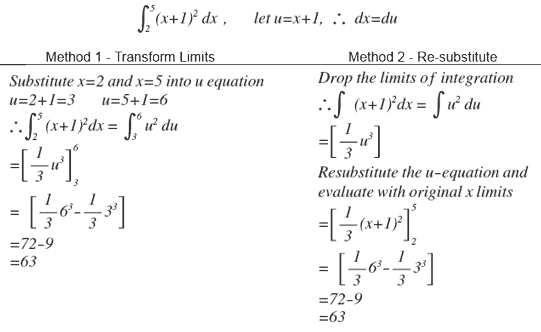
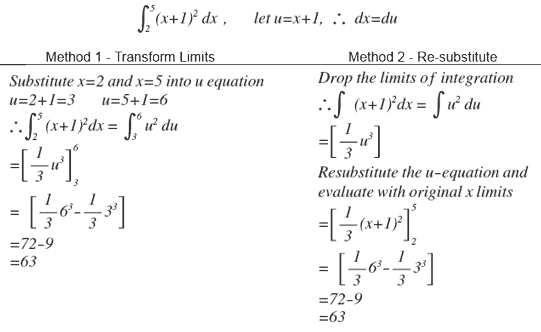
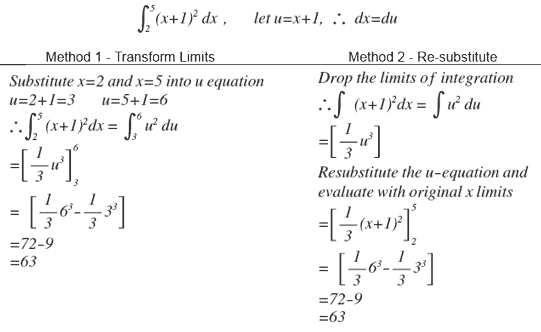
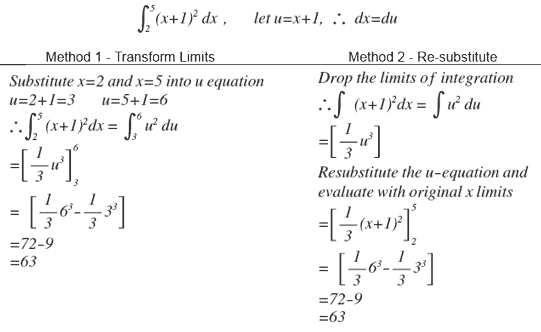
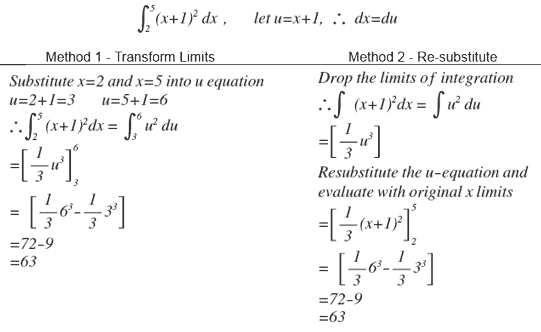
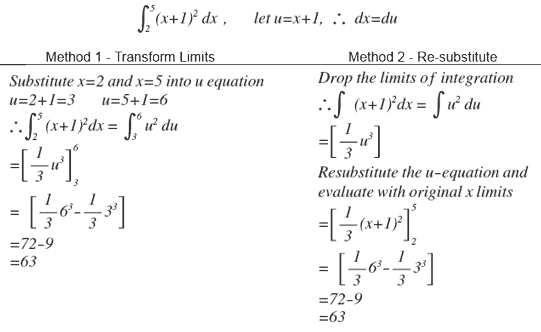
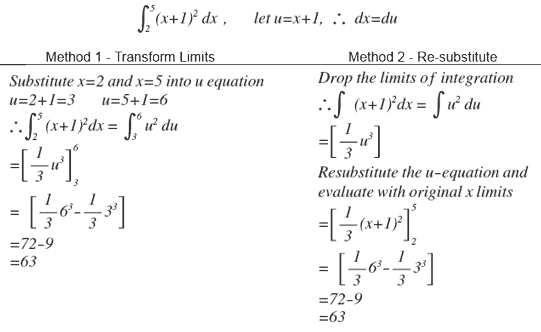
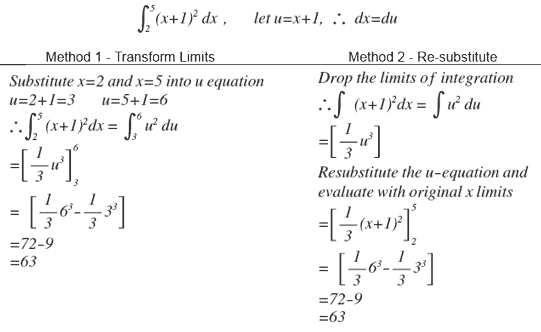
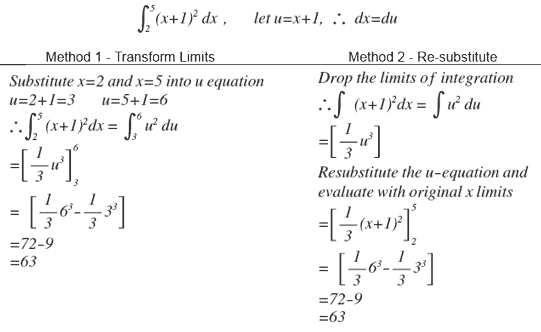
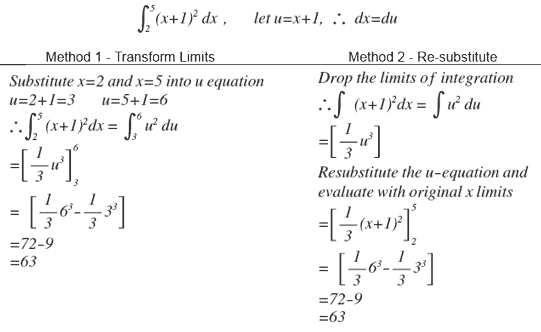
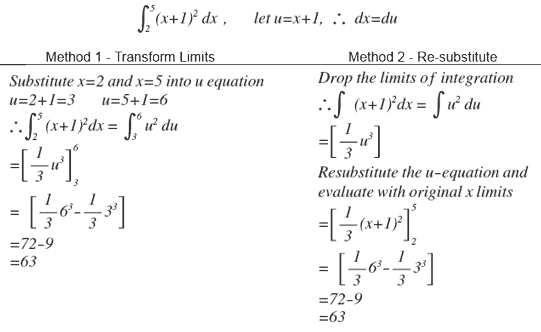
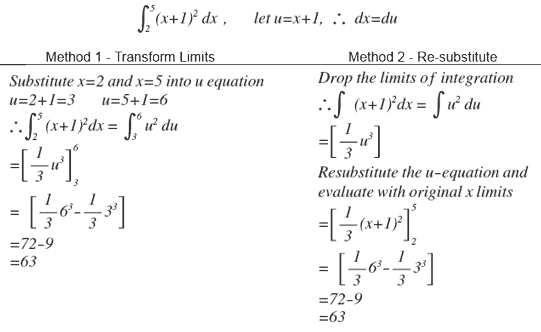
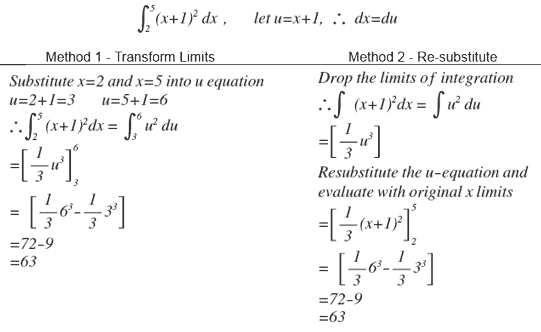
How do you determine the limits of integration after performing u-substitution?

After performing u-substitution, you determine the limits of integration by substituting the original limits into the new variable u. Then, you solve the equations to find the corresponding new limits in terms of u. Finally, you use these new limits to set up the integral with respect to u.

Are there any restrictions on the type of functions that can be solved using u-substitution?

There are no specific restrictions on the type of functions that can be solved using u-substitution, but typically it is most effective for integrals where the function being integrated is a composition of simpler functions or where there is a clear substitution that can simplify the integral. Functions with constant terms or logarithmic functions are usually not suitable for u-substitution, but other types of functions like trigonometric, exponential, or polynomial functions can often be effectively integrated using this technique.

What is the difference between definite and indefinite integrals when using u-substitution?

When using u-substitution, the main difference between definite and indefinite integrals lies in the evaluation process. For definite integrals, after making the substitution and obtaining the antiderivative in terms of u, you need to change the limits of integration from the original variable to u before evaluating the integral. This allows you to find the definite value of the integral over the specified interval. In contrast, with indefinite integrals, once you have found the antiderivative in terms of u, you then transform it back to the original variable x without needing to worry about specific limits.

How does u-substitution help simplify the process of integration?

U-substitution helps simplify the process of integration by allowing us to rewrite a complex function in terms of a simpler variable u. This allows us to simplify the integrand and potentially make it easier to integrate. By performing the substitution and then differentiating u to find du, we can rewrite the integral entirely in terms of u, making it easier to handle and leading to a more straightforward integration process.

Are there any common mistakes to watch out for when using u-substitution?

Yes, common mistakes to watch out for when using u-substitution include forgetting to differentiate u with respect to x, misidentifying which part of the integral should be substituted as u, neglecting to adjust the limits of integration when switching from x to u, and overlooking the need to replace dx with du. It's important to carefully follow the rules of u-substitution to ensure correct integration.

Can u-substitution be used to solve other types of problems besides integrals?

Yes, u-substitution can be used in differential equations, where it can help simplify the equation before attempting to solve it. By substituting variables using u-substitution, differential equations can often be transformed into more manageable forms, making it easier to find solutions.