Advanced Algebra Worksheets

Are you a high school or college student who wants to improve their skills in advanced algebra? If so, you've come to the right place! In this blog post, we will explore the benefits of using worksheets as a valuable resource for studying and practicing complex algebraic concepts. With carefully designed exercises that focus on key topics, these worksheets can help you strengthen your understanding of equations, functions, and more, allowing you to excel in your mathematics studies.

  Algebra Factoring Practice Worksheets

---

  Combining Like Terms Algebra Worksheets

---

  Standard to Vertex Form Worksheet

---

  Simple Algebra Worksheet

---

  9th Grade Algebra Math Worksheets Printable

---

What is the definition of a polynomial?

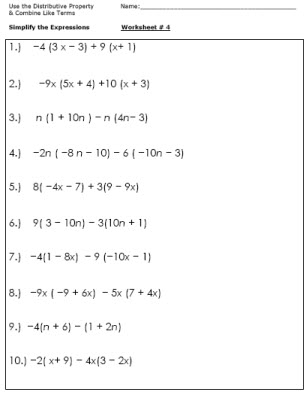
A polynomial is a mathematical expression consisting of variables and coefficients, where the variables are raised to non-negative integer powers and multiplied by the coefficients. The terms in a polynomial are typically combined using addition and subtraction, and the highest power of the variable in the polynomial is known as the degree of the polynomial.

How do you simplify expressions with rational exponents?

To simplify expressions with rational exponents, you can rewrite the expression using exponent rules. For example, if you have a term with a rational exponent like x^(3/2), you can rewrite it as the square root of x cubed or ?x^3. Similarly, if you have a term with a fractional exponent in the denominator like 1/x^(2/3), you can rewrite it as the cube root of x squared or x^(2/3). By applying these rules and manipulating the exponents accordingly, you can simplify expressions with rational exponents to a more manageable form.

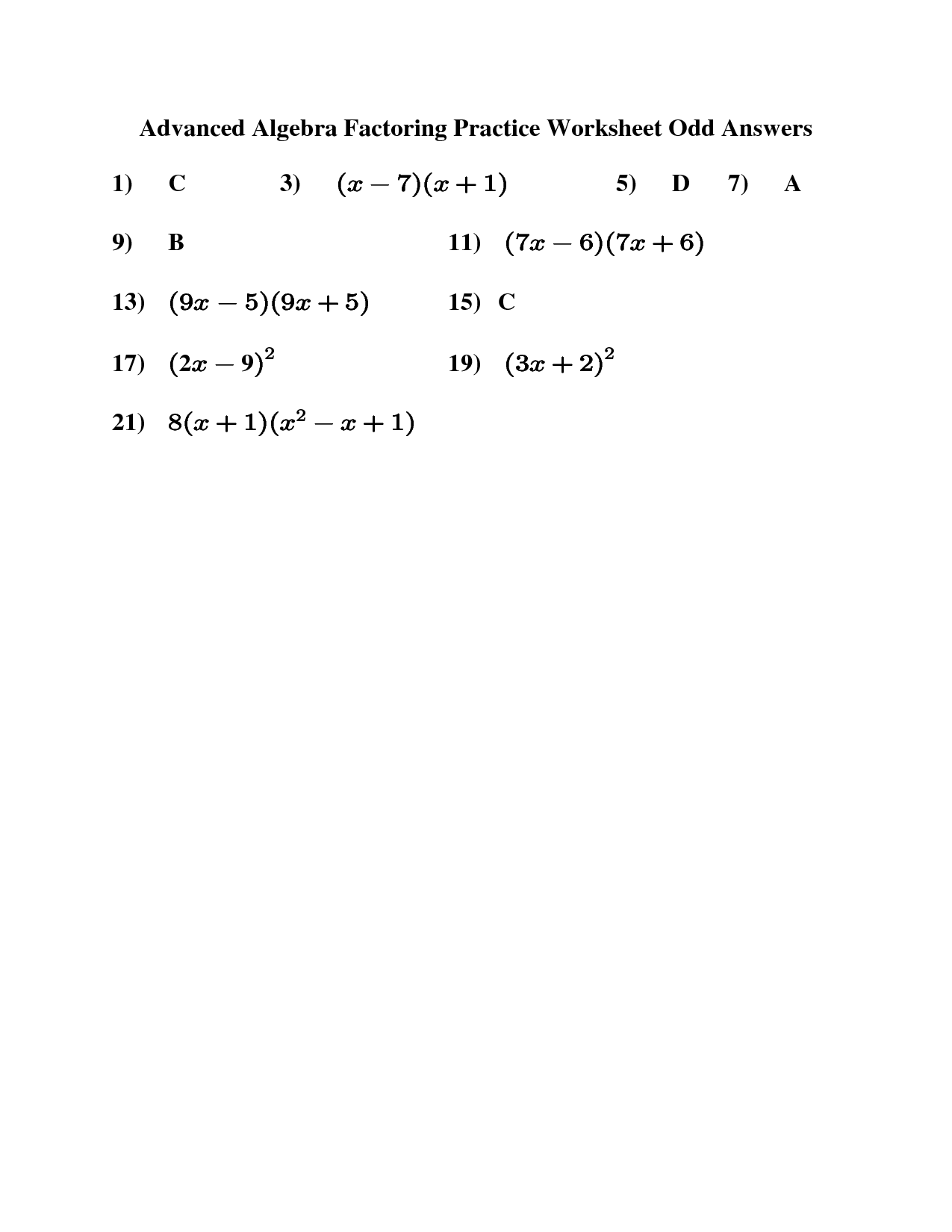
Describe the process of factoring a quadratic trinomial.

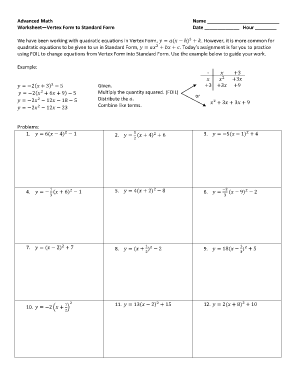
To factor a quadratic trinomial, first, check if there is a common factor that can be factored out. Then, look for two numbers that multiply to the leading coefficient (the coefficient of x^2) and add up to the middle coefficient (the coefficient of x). Next, rewrite the middle term using these two numbers and factor by grouping or using the reverse FOIL method. Finally, complete the factoring by finding the common factor between the two resulting binomials. This process helps to express the quadratic trinomial as the product of two binomials, making it easier to solve or graph.

What is the difference between an arithmetic sequence and a geometric sequence?

In an arithmetic sequence, each term is obtained by adding a constant difference to the previous term, while in a geometric sequence, each term is obtained by multiplying the previous term by a constant ratio. This means that in an arithmetic sequence, the difference between consecutive terms is constant, whereas in a geometric sequence, the ratio between consecutive terms is constant.

Explain how to find the domain and range of a function algebraically.

To find the domain of a function algebraically, look for any values of the independent variable (usually denoted as "x") that would result in division by zero, square roots of negative numbers, or any other operations that are undefined. For the range, find the possible output values of the function by substituting the domain values into the function and observing the range of resulting output values. You can also analyze the behavior of the function and consider its limits as the independent variable approaches infinity or negative infinity to determine the overall range.

Describe the steps involved in solving a system of linear equations using matrix methods.

To solve a system of linear equations using matrix methods, first, write the equations in standard form Ax = b. Then, construct the coefficient matrix A and the constant vector b. Next, calculate the inverse of matrix A if it is square by finding A^(-1). Multiply the inverse of A by vector b to solve for x, x = A^(-1) * b. If A is not square, find the transpose of matrix A and then multiply it by A to form the normal equations. Finally, solve the normal equations for x by using matrix operations.

What are rational functions and how do you identify their vertical and horizontal asymptotes?

Rational functions are functions that can be expressed as the ratio of two polynomial functions. To identify the vertical asymptotes of a rational function, find the values of the variable that make the denominator of the function equal to zero, as these will be the vertical asymptotes. To identify horizontal asymptotes, compare the degrees of the numerator and denominator polynomials. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote will be y=0. If the degrees are equal, divide the leading coefficients of the numerator and denominator to find the horizontal asymptote. If the degree of the numerator is greater, there will be no horizontal asymptote.

Explain the concept of logarithms and how they are used to solve exponential equations.

Logarithms are the inverse of exponential functions and are used to solve exponential equations by allowing us to manipulate complex exponentials into simpler forms. By converting exponential equations into logarithmic equations, we can solve for unknown variables more efficiently. Logarithms help condense large numbers into more manageable ones, making it easier to perform calculations involving very large or small numbers. Essentially, logarithms provide a way to transform exponential equations into linear form, simplifying the process of solving them.

How do you perform polynomial long division?

To perform polynomial long division, divide the leading term of the numerator by the leading term of the denominator to determine the first term of the quotient. Then, multiply the entire denominator by this term, subtract the result from the numerator, and bring down the next term. Repeat these steps until the degree of the current remainder is less than the degree of the denominator, and the process is complete, resulting in the quotient and remainder of the division.

Describe the key principles of complex numbers and how to perform operations with them.

Complex numbers consist of a real part and an imaginary part, expressed as a + bi, where 'a' and 'b' are real numbers and 'i' is the imaginary unit (?-1). The key principles of complex numbers are that they can be added, subtracted, multiplied, and divided like real numbers. Addition and subtraction involve adding or subtracting the real and imaginary parts separately. Multiplication is done by applying the distributive property and combining like terms. Division is carried out by multiplying by the conjugate of the denominator. Complex conjugates involve changing the sign of the imaginary part. Overall, operations with complex numbers require attention to both the real and imaginary parts to ensure that the calculations are accurate.